


Japanese 1:5 Soroban with kanji characters on the dividing bar representing denominations of yen.


Central Asian Schotty, 10-bead abacus


10-Bead school abacus of the kind Napoleon brought back to France from the 19th-century Russian campaign.

Here are the presentation foils included in the exhibit:




The following pages illustrate examples of abacus addition. First is how numbers are represented on the 1:4 soroban:

Next are two simple addition problems, the first one direct addition, the second using fives complements. But first I should explain the concept of "complements."
Since there are a limited number of beads on each rod, there are occasions when you can't directly add a quantity of beads. For numbers under 5, these problems are known as "fives complements." A five complement is a number that, when added to the original number, equals five. For example, the fives complements of 1,2,3 and 4 are: 4,3,2 and 1, respectively.
There are also tens complements of numbers: a number which, when added to the original number, equals ten. The tens complements of 1,2,3,4,5,6,7,8 and 9 are: 9,8,7,6,5,4,3,2 and 1, respectively.
The way that fives complements are used in addition is, instead of directly adding the number (which you can't, because there aren't enough beads left on the rod), you subtract the fives complement of the number and add a five bead from above the bar.
The way that tens complements are used in addition is, instead of directly adding the number (which you can't, because there aren't enough beads left on the rod), you subtract the tens complement of the number and add a single bead on the next rod to the left.

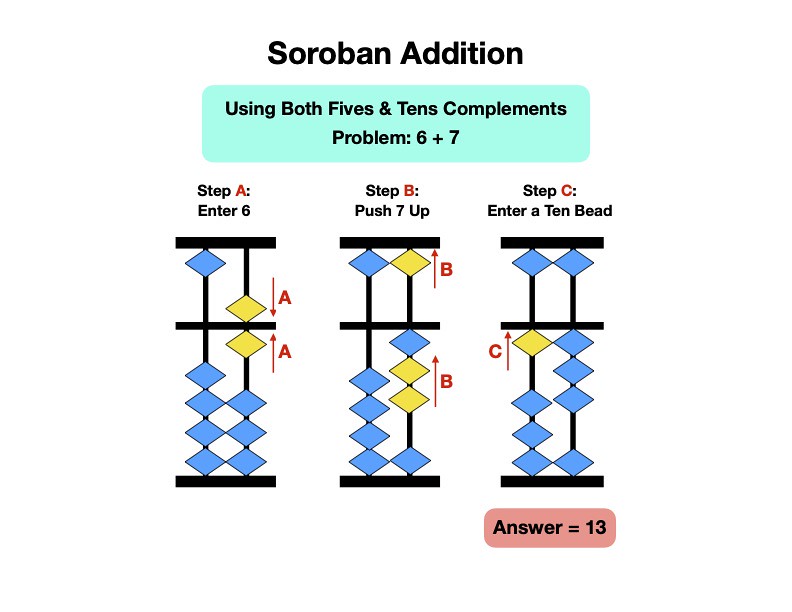
Next is a problem involving both fives and tens complements. These problems happen when you have to do a tens complement operation, but there aren't enough beads on the rod to subtract the tens complement. The shortcut method my Taiwanese abacus teacher taught was to "push the number up," as illustrated in the next foil, where in order to add 7 to 6, you "push the 7 up":
The photo below shows the classic book on Japanese soroban, by Takashi Kojima; that I first acquired in the early 1970s when I began my abacus journey. The photo is also accompanied by a contemporary Chinese-made teacher's abacus, designed so the beads stay put when hung vertically for the classroom:

A Brief Primer on Soroban Addition:
Performing addition and subtraction (the inverse of addition) efficiently on the soroban means quickly determining which kind of operation you need to perform. Though I'm not a master soroban operator, I can work my way around the beads well enough for my purposes, enough to know that I often can enter each number without consciously thinking how I did it, through rote practice. But I've since analyzed my methods, and have determined the following procedure for beginners to follow for addition on the beads. This procedure is based on Kojima's teachings, but expressed in logical steps for the western adult-brained learner.
Firstly, you do need to know the fives and tens complements.
Next are a series of questions you need to ask, in the order listed, when confronted with a single-digit addition problem:
A) Can the number be directly added? If yes: add the number, problem done. If no, go to step B.
(Examples of this kind of problem: 1 + 3 and 2 + 7)
B) Are both numbers less than five? If yes, subtract the five complement of the number and add a five bead (this is done with one swift downward move of the index finger), problem done. If no, go to step C.
(Examples of this kind of problem: 4 + 3 and 3 + 2)
C) Can the tens complement be directly subtracted? If yes, subtract the tens complement and add a bead on the next rod to the left, problem done. If no, go to step D.
(Examples of this kind of problem: 3 + 8 and 9 + 6)
D) Push the number up, then add a bead on the next rod to the left.
(Examples of this kind of problem: 5 + 9 and 7 + 6)
There is one more complication. Sometimes, when dealing with multi-digit numbers, when doing a tens complement problem and you need to add the single bead on the next rod to the left, if that rod already holds a value of either 4 or 9, you can't directly add that single bead. If the rod has a 4, you need to swipe down the five bead and all four ones beads (essentially doing a fives complement operation in order to add the single bead). If the rod has a value of 9, you have to clear the nine out and add the single bead to the next rod to its left.
I should also mention that when adding multi-digit numbers, you do so from left to right, like the order in which you would write or speak them. This is more efficient than the right-to-left paper method we were taught in school.
I know that for the rank beginner this all sounds complicated. But what I've described thus far is all you need to know to do addition problems involving numbers of any length. You do them one digit at a time, from left to right, using the rules I've described above.
Know also that I will be touching on this method in more depth in an upcoming video on my YouTube channel.
The timing of this post is most interesting. Just this past weekend I was watching a motorcycle race. As the race progressed, the announcers were trying to calculate the projected overall season standings of the riders. Such projections were based on the total number of points the riders had already acquired thus far in the season leading up to this particular race, combined with the points the riders stood to gain based on their projected placing at the end of the race. At one point, one of the announcers noted that it was probably time to break out the abacus in order to better keep up with the ever changing math involved.
ReplyDeleteNaturally my first thought upon hearing this was of yourself Joe, as we've discussed your interest in the abacus many times on Typewriter Club Live. Then my thoughts turned to wondering just what the odds might be that someone would bring up the abacus under such circumstances in this day and age. Of course the fact that my lifelong personal interest in dirt bike racing would somehow intersect with my current passion for typewriters via the typewriter community and one member of that community in particular with his passion for the abacus is more than mind-boggling itself.
Side Note:
The particular form of motorcycle racing that I was watching is known as supercross. Supercross is a spin-off of motocross racing. Both events are forms of dirt bike racing. Rather than competing on tracks set up in wide open spaces outdoors as in motocross, supercross has the riders competing on dirt tracks set up in various stadiums designed for other sporting events such as football and baseball stadiums. Ironically, one of the best racers in the country at the highest level of dirt bike racing (be it supercross or motocross), is Rio Rancho's Jason Anderson. Had the race announcers made the proper predictions and used an abacus correctly, they would have known that Jason Anderson would currently sit in 2nd place in the overall standings.
Wonderful exhibit, Joe! Thanks for sharing with your fans too far away to visit. My mom grew up using an abacus in school. She still has two of them, a small portable one and a much larger one made of marble, a beautiful shelf queen nowadays. She did try to teach us to calculate with it, but I have since forgotten. Thanks for the refresher!
ReplyDeleteAfter seeing your video, I pulled out one of the abacuses I recently obtained in a box of miscellaneous office supplies and used your method. It took a few times, but today I managed to add two numbers from a receipt and get the correct answer!
ReplyDeleteSuch an wonderful blog. Your blog is so informative and interesting to me and. I am very impressed by your writing skill. Thanks for sharing this blog and good information. Now it's time to avail african gowns for ladies for more information.
ReplyDelete